Electromagnetic (EM) radiation is a periodically changing or oscillating electric field propagating in a certain direction with a magnetic field oscillating at the same frequency but perpendicular to the electric field [1].
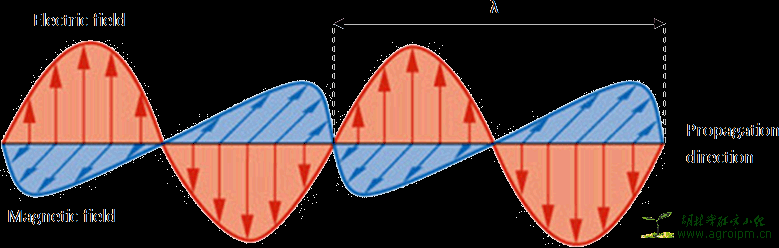
Figure 1: A schematic representation of EM radiation. The wavelength is represented by λ. Adapted from [2].
EM radiation may be considered as a traveling wave or as a stream of massless elementary particles, often called photons. As a wave, it can be characterized by its wavelength λ (the length of one wave), its frequency ν (the number of vibrations per unit time) and its wavenumber k (the number of waves per unit length) [3]. The velocity for EM-waves proves to be a constant for the medium in which the waves are propagating, that is 3 · 108 m/s in vacuum. It can be easily seen that
the wavelength being inversely proportional to the frequency. Using the quantum character of EM radiation, there is the relation
where E is the photon energy and h is Plank’s constant [4]. Each quantum of radiant energy or photon, has an energy proportional to its frequency ν [5]. By this means, a photon with a higher frequency contains more energy.
The EM spectrum can be divided into several regions differing in frequency only. It extends from gamma (ν≥1020Hz) and X-rays (3·1016≤ν<1020Hz) to radio frequencies (ν<0.003Hz) and includes ultraviolet (7.5·1014≤ν<3·1016Hz), visible (4·1014≤ν<7.5·1014Hz) and infrared (IR) regions (0.003≤ν<4·1014Hz). In vacuum, IR radiation has a wavelength between ~780 nm and 1 mm, spanning three orders of magnitude and can be divided in Near IR, Short wave IR, Mid wave IR, Long wave IR, and Far IR. An evident and comprehensive illustration of the EM spectrum can be found on Wikipedia.
The basic building blocks of the ‘common’ matter in the universe are atoms, and combinations of atoms are called molecules. The energy of a molecule consists of translational, rotational, vibrational and electronic energy [3].
Translation energy of a molecule is associated with the movement of the molecule as a whole, for example in a gas. Rotational energy is related to the rotation of the molecule, whereas vibrational energy is associated with the vibration of atoms within the molecule. Finally, electronic energy is related to the energy of the molecule's electrons.
Like radiant energy, the energy of a molecule is quantized too and a molecule can exist only in certain discrete energy levels. Within an electronic energy level a molecule has many possible vibrational energy levels. To raise the electronic energy state of a molecule from the ground state to the excited state will cost more energy than to raise the vibrational energy state. A simplified representation of the quantized electronic and vibrational energy levels of a molecule can be found in figure 2.
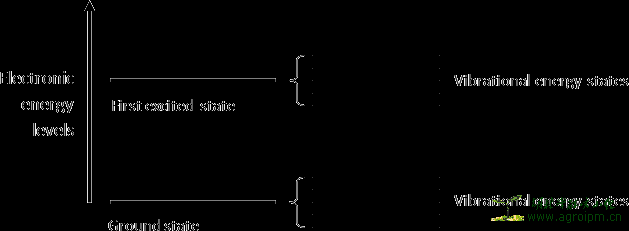
Figure 2: A schematic representation of the quantized electronic and vibrational energy levels of a molecule. It is clear that to raise the electronic energy state of a molecule from the ground state to the excited state will cost more energy than to raise the vibrational energy state.
An example of an electronically excited atom comes by considering the hydrogen atom. The ground state of the hydrogen atom corresponds to having the atom's single electron in the lowest possible orbits. By giving the atom additional energy, the electron is able to move into a higher orbit. Now, we deem the hydrogen atom to be in its excited state [6].
The vibrational energy of a molecule is not determined by the orbit of an electron but by the shape of the molecule, the masses of the atoms and, eventually by the associated vibronic coupling1). In the study of molecular vibrations I can start with a classical model of the molecule where the nuclei are represented by mathematical points with mass. The forces that hold the molecule together are assumed to be similar to those exerted by massless springs. Each mass requires three coordinates to define its position, such as x, y, and z in a Cartesian coordinate system. As a result it has three independent degrees of freedom of motion. If there are N atomic nuclei in the molecule, there will be a total of 3N degrees of freedom of motion for all the nuclear masses in the molecule. After subtracting the translational and rotational degrees of freedom from the total 3N degrees of freedom, we are left with 3N – 6 internal degrees of freedom for a nonlinear molecule and 3N – 5 internal degrees of freedom for a linear molecule. These are the so-called normal modes of vibrations and they result in specific natural vibrational frequencies for different molecular configurations. The typical frequencies of these vibrations match the frequency of IR radiation (!).
For example, simple diatomic molecules have only one bond allowing only stretching vibrations. More complex molecules may have many bonds, and vibrations can be conjugated. The atoms in a CH2 group, commonly found in organic compounds, can vibrate in six different ways; symmetrical and antisymmetrical stretching, scissoring, rocking, wagging and twisting.
Now that I have discussed the energy level structure of molecules, I will address the interaction of these molecules with EM waves. These interactions are at the very heart of IR spectroscopy.
Roughly, there are five possible effects of interaction between radiation and molecules. These are scattering, absorption, and emission. [8]. Absorption is the process by which the energy of a photon is taken up by the matter, and this process plays a key role in IR spectroscopy. There are several types of physical processes that could lie behind absorption, depending on the quantum energy of the particular frequency of EM radiation. For example, high energetic ultraviolet (UV) radiation can cause ionization and visible light usually causes electron transitions. As told before, the energy levels for all physical processes at the atomic and molecular levels are quantized, and if there are no available quantized energy levels with spacings which match the quantum energy of the incident radiation, then the material will be transparent to that radiation. In view of IR spectroscopy, I will now focus on the absorption of IR radiation by matter.
IR radiation does not have enough energy to induce electronic transitions as seen with UV and visible light. Absorption of IR is restricted to excite vibrational and rotational states of a molecule.
Even though the total charge on a molecule is zero, the nature of chemical bonds is such that the positive and negative charges do not necessarily overlap in this case. Such molecules are said to be polar because they possess a permanent dipole moment. For a molecule to absorb IR, the vibrations or rotations within a molecule must cause a net change in the dipole moment of the molecule. The alternating electrical field of the radiation interacts with fluctuations in the dipole moment of the molecule. If the frequency of the radiation matches the vibrational frequency of the molecule then radiation will be absorbed, causing a change in the amplitude of molecular vibration. The result of IR absorption is heating of the matter since it increases molecular vibrational energy. Molecular vibrations give rise to absorption bands throughout most of the IR region of the spectrum. The far IR, lying adjacent to the microwave region, has low energy and may be used for rotational spectroscopy.
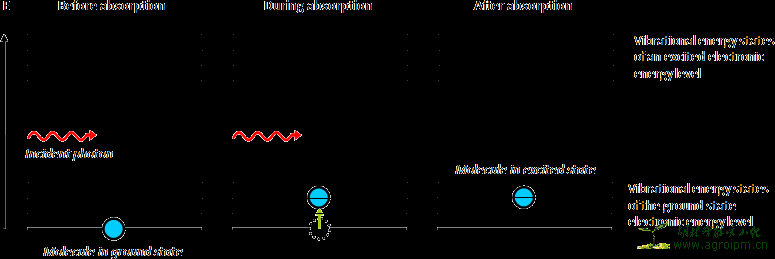
Figure 3: Energy levels of a molecule during the absorption of a photon. Photons with specific energies will be absorbed by the molecule if this energy is equal to the difference between the energy levels; this is when the frequency of the IR radiation matches the vibrational frequency of the molecule. Before absorption of the incident photon the molecule is in the ground state. After absorption the molecule is in an excited vibrational state but still in the ground state of the electronic energy level. Of course the molecule does not remain in this excited state forever. The energy absorbed by a molecule is rapidly dissipated: it will be transformed into kinetic energy as result of collisions or released again as photon.
以下文章转载自小木虫 笑玄97 天然产物的研究发现之路漫长而修远,你我求索于其中孜孜不悔;顾盼回首,我们究竟走了多远?本帖所述文...

反相柱填料主要以硅胶为基质,在其表面键合非极性的十八烷基官能团(ODS)称为C18柱,其它常用的反相柱还有C8,C4,C2和苯基柱等。另外还有离...

以下文章转载自小木虫 swaucq 文献 Hamamoto, H.; Urai, M.; Ishii, K.; Yasukawa, J.; Paudel, A.; Murai, M.; Kaji, T.; Kuranaga, T.; Hamase, K.; Katsu, T.; Su, J.; Adachi, T...
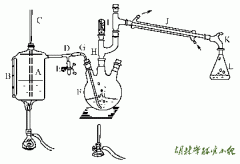
水蒸气蒸馏(steam distillation)是分离纯化液体或固体 化合物 的常用方法之一。 (1)水蒸气蒸馏的 应用 范围和条件 水蒸气蒸馏适用于以下情况:...
 微信扫码 关注我们
微信扫码 关注我们

24小时咨询热线

移动电话